Welcome to the measurement worksheets page at Math-Drills.com where you can measure up, measure down or measure all around! This page includes Measurement worksheets for length, area, angles, volume, capacity, mass, time and temperature in Metric, U.S. and Imperial units.
Measurement concepts and skills give students the ability to perform tasks related to everyday life. Length, area, volume, capacity, mass, time and temperature are measurement concepts that people are exposed to everyday. Students begin using non-standard units such as their own height and progress to using standard measurement units. Being able to recognize and use for comparison, common measurement units such as the metre or foot, allows students to use their estimation skills to help them solve problems in measurement. Measurement tools enable students to learn hands-on and develop a deeper understanding of measurement concepts.
Most Popular Measurement Worksheets this Week


Reading and Converting Temperature Worksheets
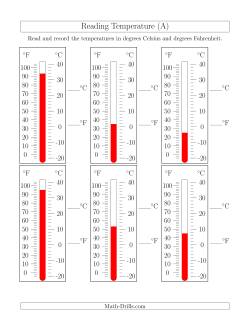
Reading temperatures from a thermometer is one of those everyday skills that is transferable to other situations. Sure, students could just ask their smart device about the temperature, but that only tells them the temperature at the nearest weather station, not where they're standing. Wouldn't it be more exciting to look at a thermometer in their own yard? And what if they are working at a solar power farm (or elsewhere) in the future and have other meters and gauges to read? Their skill in reading thermometers will help introduce them to negative numbers and be useful for reading other types of meters and gauges in the future.
Millions of people live near the U.S./Canada border, so it is inevitable that once in a while, those people are going to hear or see the temperature in a less familiar unit. Perhaps traveling to another country has one baffled about the forecast. Having a knowledge of some simple equivalents (like the freezing point is 0 degrees Celsius and 32 degrees Fahrenheit) and the ability to convert between C and F will not only help in determining the temperature in a familiar form, but will also help students build math skills that are useful in other situations. The temperature conversion guide gives a summary of the formulas used and some whole number equivalents to learn. There are several practice sheets for converting from °C to °F, from °F to °C, and a couple with both conversions mixed up.
Measuring and Converting Within Measurement Systems

Measuring length is so much more interesting if you can send students out with rulers and have them measure items in their environment. What is the width of the textbook? the classroom? the school? Have you ever met a student who didn't enjoy using a measuring wheel (you know the one that clicks every time you've traveled a yard or a meter)? How do you know they've measured things correctly though? Well, you might need something like we've provided below. You can also compare students' measurements of the same objects to see if they got the same measurement. Let's say, you had 20 students measure the height of the doorway. You should get 20 very similar answers (unless they are the sharing type then you'll get exactly the same answers) and any different answers can be quickly identified. IMPORTANT: When printing, select "Actual Size" or your measurements may be off.
Just like regrouping when adding, sometimes it is useful to regroup when measuring. If you have too many feet, it might be easier to express your number in yards or miles, for example.
Which unit to use with mass depends a lot on the quantity and what you want to communicate. Whatever your reason for converting mass measurements, these worksheets are likely able to help you take a weight off your shoulders.
The liquid measurement worksheets include gills because this is the key unit that results in more fluid ounces in an Imperial gallon than in a U.S. gallon. You can learn more about gills in our liquid measurement conversion guide.
Even though Imperial and U.S. Customary units may sound the same, they aren't always the same amount. For example, there are 3.785 litres in a U.S. gallon and 4.546 litres in an Imperial gallon. Sometimes there are also different definitions for units like the gill used in liquid measurements. In the U.S., there are 4 fluid ounces in a gill and in the Imperial System, there are 5 fluid ounces in a gill.
Converting between Metric units is really an exercise in multiplying and dividing by powers of ten. Each of the converting worksheets in this section includes a "Conversion Line" that includes the prefixes, symbols and powers. It can be used to figure out how many "steps" are required to convert from one unit to another and what operation must be used. For example, when converting from millimeters to kilometers, students would determine that it takes six steps to the left in the direction of the division sign to get from milli to kilo on the conversion line. Depending on which method they are taught, this could involve dividing by 10 six times, dividing by 106 or "moving the decimal" six places to the left. For squared units, each step counts as 100 or 102 and for cubic units, each step counts as 1000 or 103. For more details, please see the Metric system conversion guide.
-
Converting Between Millimeters and Centimeters ✎ Converting Between Centimeters and Meters ✎ Converting Between Millimeters and Meters ✎ Converting Between Millimeters, Centimeters and Meters ✎ Converting Between Meters and Kilometers ✎ Converting Between Millimeters, Centimeters, Meters, and Kilometers ✎ Converting Between Nanometers, Micrometers, Millimeters and Centimeters ✎
-
Converting Between Milliliters and Liters ✎ Converting Between Microliters, Milliliters, Centiliters and Liters ✎ Converting Between Milliliters, Centiliters, Liters and Kiloliters ✎ Converting Between Milliliters, Liters, and Kiloliters ✎ Converting Between Liters, Kiloliters, Megaliters and Gigaliters ✎
The Metric or SI system uses thin spaces for thousands separators and spells metres and litres with -re rather than -er. This section is mainly for students in English Canada, however, anyone who uses spaces for thousands separators might like these worksheets. This section is very similar to the previous section except for the differences in number formats and spelling.
-
Converting Between Millimetres and Centimetres (SI number format) ✎ Converting Between Centimetres and Metres (SI number format) ✎ Converting Between Millimetres and Metres (SI number format) ✎ Converting Between Millimetres, Centimetres and Metres (SI number format) ✎ Converting Between Metres and Kilometres (SI number format) ✎ Converting Between Millimetres, Centimetres, Metres, and Kilometres (SI number format) ✎ Converting Between Nanometres, Micrometres, Millimetres and Centimetres (SI number format) ✎
-
Converting Between Millilitres and Litres (SI number format) ✎ Converting Between Microlitres, Millilitres, Centilitres and Litres (SI number format) ✎ Converting Between Millilitres, Centilitres, Litres and Kilolitres (SI number format) ✎ Converting Between Millilitres, Litres, and Kilolitres (SI number format) ✎ Converting Between Litres, Kilolitres, Megalitres and Gigalitres (SI number format) ✎
-
Converting Between Square Millimetres and Square Centimetres (SI number format) ✎ Converting Between Square Centimetres and Square Metres (SI number format) ✎ Converting Between Square Millimetres, Square Centimetres and Square Metres (SI number format) ✎ Converting Between Square Metres, Square Hectometres and Square Kilometres (SI number format) ✎
Similar to the previous two sections; however, these worksheets use dots for thousands separators and commas for decimals. This is often found in languages other than English. Metres and litres are spelled with -re as you would find in many countries outside of the U.S.
-
Converting Between Millimetres and Centimetres (Euro number format) ✎ Converting Between Centimetres and Metres (Euro number format) ✎ Converting Between Millimetres and Metres (Euro number format) ✎ Converting Between Millimetres, Centimetres and Metres (Euro number format) ✎ Converting Between Metres and Kilometres (Euro number format) ✎ Converting Between Millimetres, Centimetres, Metres, and Kilometres (Euro number format) ✎ Converting Between Nanometres, Micrometres, Millimetres and Centimetres (Euro number format) ✎
-
Converting Between Square Millimetres and Square Centimetres (Euro number format) ✎ Converting Between Square Centimetres and Square Metres (Euro number format) ✎ Converting Between Square Millimetres, Square Centimetres and Square Metres (Euro number format) ✎ Converting Between Square Metres, Square Hectometres and Square Kilometres (Euro number format) ✎
Converting Between Measurement Systems Worksheets

Converting between Metric and U.S. customary units can be accomplished in a number of ways and usually takes a little knowledge of fractions and/or decimals. Most commonly, students will use a formula to convert and round the values. You may like our converting inches and centimeters with rulers worksheets for students who have difficulty with manipulating the numbers and formulas and need an easier method.
-
Converting Between U.S. Inches and Centimeters Converting Between Meters and U.S. Feet and Yards Converting Between U.S. Miles and Kilometers Converting Between U.S. Feet and Kilometers and Meters and U.S. Miles Converting Between Metric and U.S. Length Units Converting Between Metric and U.S. Length Units including ft/km and m/mi
Measuring Angles, Rectangles and Triangles
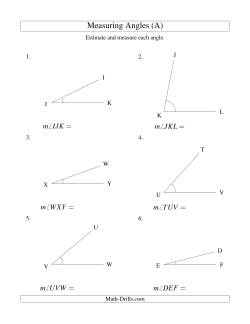
If they are available, full round protractors help students to recognize that measuring angles is the same as measuring sections of a circle. They also makes it much easier and precise to measure reflex angles.
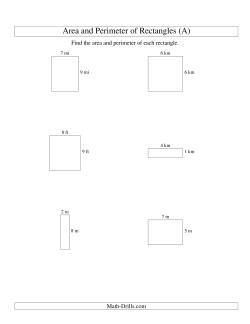
Rectangles are fairly straight-forward polygons to measure since it is easy to find rectangles that use whole numbers. Rectangles are generally used when students first learn about perimeter and area and it is an opportune time to teach students that units are an essential part of any measurement. Without units, numbers are meaningless. Get your students into the habit of expressing all of their measurements with the correct units before they learn how to measure other polygons. Especially make sure they know that area is always expressed with squared units.
If a student is just starting to learn about perimeter and area, a few hands-on activities to learn the concepts is a good idea. Have them use square tiles to cover an area, have them paint a piece of paper and see how much paint is required. Create rectangles with straws and pipe cleaners and fill with square tiles to differentiate between perimeter and area. See if there are differently shaped rectangles that will hold the same number of square tiles.
-
Calculate the Area and Perimeter of Rectangles from Side Measurements (Smaller Whole Numbers) Calculate the Area and Perimeter of Rectangles from Side Measurements (Larger Whole Numbers) Calculate the Area and Perimeter of Rectangles from Side Measurements (Decimal Numbers) Area of Rectangles (grid form)
-
Calculate the Side and Area Measurements of Rectangles from Perimeter and Side (Smaller Whole Numbers) Calculate the Side and Area Measurements of Rectangles from Perimeter and Side (Larger Whole Numbers) Calculate the Side and Area Measurements of Rectangles from Perimeter and Side (Decimal Numbers) Calculate the Side and Perimeter Measurements of Rectangles from Area and Side (Smaller Whole Numbers) Calculate the Side and Perimeter of Rectangles from Area and Side Measurements (Larger Whole Numbers) Calculate the Side and Perimeter of Rectangles from Area and Side Measurements (Decimal Numbers) Calculate the Side Measurements of Rectangles from Perimeter and Area (Smaller Whole Numbers) Calculate the Side Measurements of Rectangles from Perimeter and Area (Larger Whole Numbers) Calculate the Side Measurements of Rectangles from Perimeter and Area (Decimal Numbers) Calculate Various Rectangle Measurements (Smaller Whole Numbers) Calculate Various Rectangle Measurements (Larger Whole Numbers) Calculate Various Rectangle Measurements (Decimal Numbers)
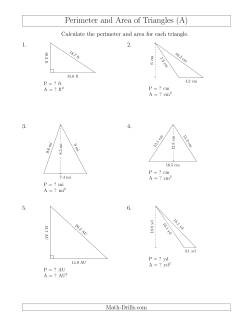
If you want students to understand the triangle area formula, you might want to study parallelograms and rectangles first. Once students get how area is calculated for rectangles and parallelograms, they simply need to cut parallelograms and rectangles in half diagonally to get related triangles. They should quickly see that the area of a triangle is simply half of the area of the related quadrilateral.
-
Calculate the Area and Perimeter of Acute Triangles Calculate the Area and Perimeter of Acute Triangles (Rotated Triangles) Calculate the Area and Perimeter of Right Triangles Calculate the Area and Perimeter of Right Triangles (Rotated Triangles) Calculate the Area and Perimeter of Obtuse Triangles Calculate the Area and Perimeter of Obtuse Triangles (Rotated Triangles) Calculate the Area and Perimeter of Acute and Right Triangles Calculate the Area and Perimeter of Acute and Right Triangles (Rotated Triangles) Calculate the Area and Perimeter of Various Triangles Calculate the Area and Perimeter of Various Triangles (Rotated Triangles)
-
Calculate Angles of a Triangle Given Other Angle(s) Calculate the Perimeter and Height Measurements of Triangles Calculate the Area and Height Measurements of Right Triangles Calculate the Base and Height Measurements of Triangles Calculate Various Measurements of Triangles Calculate the Perimeter and Area from Side Measurements of Triangles (Heron's Formula)
Measuring Other Polygons
-
Calculate the Area and Perimeter of Trapezoids (Smaller Numbers) Calculate the Area and Perimeter of Trapezoids (Larger Numbers) Calculate the Area and Perimeter of Trapezoids (Even Larger Numbers) Calculate the Area and Perimeter of Trapezoids (Larger Still Numbers) Calculate the Area and Perimeter of Isosceles Trapezoids Calculate the Area and Perimeter of Right Trapezoids Calculate the Area and Perimeter of Scalene Trapezoids
-
Calculate the Area and Perimeter of Trapeziums (Smaller Numbers) Calculate the Area and Perimeter of Trapeziums (Larger Numbers) Calculate the Area and Perimeter of Trapeziums (Even Larger Numbers) Calculate the Area and Perimeter of Trapeziums (Larger Still Numbers) Calculate the Area and Perimeter of Isosceles Trapeziums Calculate the Area and Perimeter of Right Trapeziums Calculate the Area and Perimeter of Scalene Trapeziums
The shapes are mixed up on the worksheets in this section. These area and perimeter worksheets would be best suited to students who have mastered finding the areas of triangles, rectangles, parallelograms, and trapezoids.
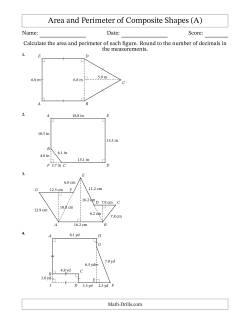
Compound or composite shapes are polygons that are made up of simpler shapes such as triangles and rectangles. Calculating the area of composite shapes involves calculating the areas of the simpler shapes. Calculating the perimeter involves determining which sides are on the outside of the composite shape and adding up their lengths. Sometimes this requires further calculations of hypotenuses and arc lengths or deriving side measurements from other measurements. For the more technical, assume that any horizontal or vertical measurement is precise and other measurements are derived and often rounded. This affects the number of significant digits, of course, but these worksheets take a simpler approach and ask students to round their answers to the number of decimal places used in the measurements.
Measuring Circles
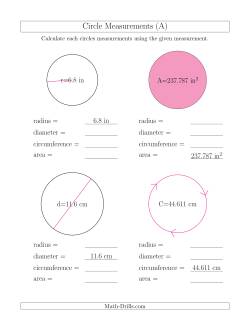
Radius, diameter, circumference and area are all related measurements; you only need one of them to find the remaining measurements. Diameter and radius are the simplest ones because the diameter of a circle is twice the radius and, conversely, the radius is half the diameter. To calculate between radius/diameter and circumference/area, you need to use π (pi). Depending on your accessibility to calculators or computers, you may use many digits of pi in the calculation or just a few. Often, people without calculators use an estimate of pi (3 or 3.14). Just for fun we made a worksheet with pi to 100,000 decimal places. The calculations on the worksheets below use a fairly precise version of pi; you may have to adjust the answers if you use more rounded versions of pi.
-
Calculate All Circle Measurements Calculate the Area & Circumference from Radius Calculate the Area & Circumference from Diameter Calculate the Area & Circumference Calculate the Radius & Diameter from Area Calculate the Radius & Diameter from Circumference Calculate the Radius & Diameter Calculate the Area & Circumference (old)
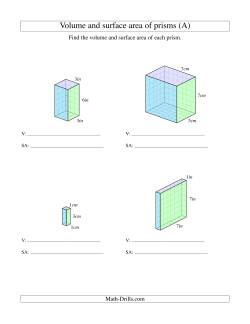
Measuring Three-Dimensional Forms